Everything from the air that you breathe to the burning cores of distant stars, all known matter in the universe is composed of atoms. Yet after generations of scientific innovation, it is still impossible to view the subatomic particles that make up these universal building blocks. That’s why this year’s Nobel Prize in Physics is being awarded to Anne L’Huillier, Pierre Agostini, and Ferenc Krausz—for observing the unobservable with attosecond spectroscopy.
First, here’s some perspective. It takes the Earth approximately 365 days to orbit the Sun. In comparison, the time it takes an electron to orbit the nucleus of its atom is considerably faster. The nucleus moves in the timescale of a femtosecond (one-quadrillionth of a second), but their electron companions are a lot smaller and a lot speedier. In reference to electrons, quantum states like speed or position shift in the timescale of an attosecond (better, one-quintillionth of a second or 0.000000000000000001 seconds). The duration of an attosecond is, in fact, “so short that there are about as many attoseconds in a single second as there have been seconds in the entire history of the universe” (Garisto, 2023).
When taking an image of a fast-moving object, in order to prevent a blurry picture, photographers use strobe lighting and a shutter speed that’s quicker than the object itself. Though no camera yet exists powerful enough to actually take a photo of an electron, just like in photography, scientists would still need to produce pulses of light faster than an electron to accurately visualize its behavior. In 1999, Ahmed Zewail won the Nobel Prize in Chemistry for a similar feat: he produced flashes of light lasting a few femtoseconds. At this speed, with his “world’s fastest camera,” Zewail was able to study the spectral emissions of chemical reactions in “slow motion” (Browne, 1999). But electrons move in attoseconds, not in femtoseconds. Zewail had pushed technology to its limit; attosecond pulses of light would either require groundbreaking new technology or an innovative and ingenious approach to producing light.
Ultraviolet Harmonics in Infrared Lasers
Light (as we understand it today) is created when an electron absorbs energy, gets excited, and then falls back to its original energy state consequently releasing its gained energy in the form of light. This is where our first Nobel Laureate, Anne L’Huillier, comes in. In the 1980s, L’Huillier and her colleagues irradiated various noble gasses with infrared photon beams (Aka laser beams). They did this through multiphoton ionization processes (MPI), in which an atom becomes ionized, losing an electron, by absorbing multiple low-energy photons rather than a singular high-energy photon (Ferray et al., 1988). L’Huillier's team noticed that in these gasses, expressly distinct in argon, the outgoing beam fired through the gas not only consisted of the laser’s original frequency but also a series of higher frequencies that were proportional to the original wavelength of the laser (Davour, 2023). This is known as high-harmonic generation (HHG), and it has become the standard for observing electrons through methods of spectroscopy.
As the infrared laser passes through an argon gas atom, the electromagnetic field binding the electrons to the atom becomes disturbed. An electron can now escape the pull of the nucleus through quantum tunneling, leaving a positively charged ion behind. However, in what is the phenomenon called recollision (see Figure 1), due to the laser’s oscillating frequency, the electromagnetic force drags back the electron towards its atom. When the electron returns to the atom, in order to ionize, all of its extra energy gained from its journey needs to be released. This energy is released in a single photon with a higher frequency than that which made up the original laser pulse (Davour, 2023). Because the infrared laser is being passed through the gas by means of MPI, the cycle is able to repeat without the electrons becoming permanently detached from their atoms, and thus the number of emitted photons is able to remain relatively constant rather than decreasing as would be expected from a normal laser pulse.
Figure 1
Recollision Model of an Electron and its Atom
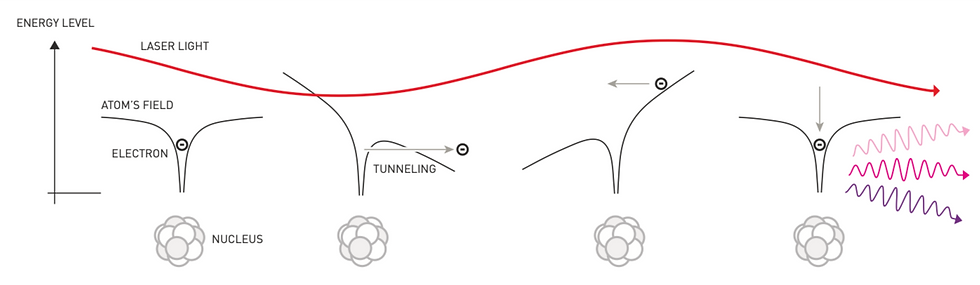
(Jarnestad & The Royal Swedish Academy of Sciences, 2023)
As stated before, the observed higher frequencies of the photons were proportional to the original infrared laser at integer multiples (specifically, odd multiples). This is known as an overtone (see Figure 2), which essentially has a similar effect to that of “repeating the same note on a piano, but at higher octaves” (Castelvecchi & Sanderson, 2023). And it is just like in a piano, where a higher frequency corresponds to a higher pitch, in the electromagnetic spectrum, a higher frequency corresponds to a wavelength with higher energy. The infrared laser is eloquently overlain with extreme ultraviolet light. This ultraviolet overtone is furthermore defined as a harmonic wave because it is seamlessly proportional to the infrared at integer multiples. L’Huillier found these ultraviolet overtones to be fairly constant from the 5th to the 33rd harmonic generation (Ferray et al., 1988). Importantly, this is the very first observation of HHG with a clear plateau, and what’s more, is that each pulse is mere attoseconds in size. Science now has a method to produce attosecond pulses.
Figure 2
Fundamental Standing Waves with Harmonic Overtones
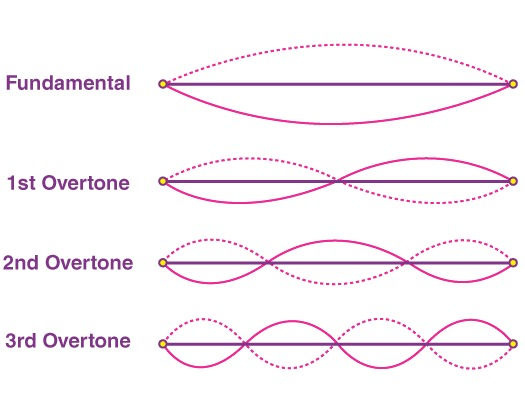
("Overtones," n.d.)
Constructive and Destructive Interference Times Attosecond Clocks
For the attosecond pulses to become a practical application for experimenters, a measurement system first needs to be made. One way to measure the length of ultrashort pulses is with a technique called FROG (frequency-resolved optical gating), in which the unknown pulse is combined with a time-delayed reference pulse. FROG is, however, typically employed for measuring femtosecond pulses, so in 2001, a group led by Pierre Agostini developed a similar approach called RABBIT (reconstruction of attosecond beating by interference of two-photon transitions), which would resolve in the attosecond domain (Paul et al., 2001). Yes, scientists love their animal-named acronyms. RABBIT works by splitting the initial infrared laser in two directions, with one beam passing through the argon gas, producing the “train” of attosecond pulses, and the other set on a calculated time delay. After the separate beams run their course, they’re recombined, and the spectral emission is examined. With this technique, Agostini’s group was able to accurately produce and measure a “string of laser pulses, each lasting 250 attoseconds” (Wood, 2023).
This is possible because of the math that describes waves. “Once they[Agostini’s group] understood which overtones to expect”—based on the contributions of L’Huillier’s work—“they worked out ways to overlay them so that they added up to a new wave: one with peaks surging on the attosecond scale” (Wood, 2023). When adding waves of different frequencies together, if their peaks coincide at the same points, the resultant frequency will be of greater intensity, having an increased amplitude. This is constructive interference, and when the troughs of different waves coincide, the result is destructive interference, having a diminished amplitude. When the ultraviolet overtones of the gas were put in phase with each other, the pulses became concise and of consistent distances apart (see Figure 3).
Figure 3
Constructive and Destructive Interference Producing Attosecond Pulses
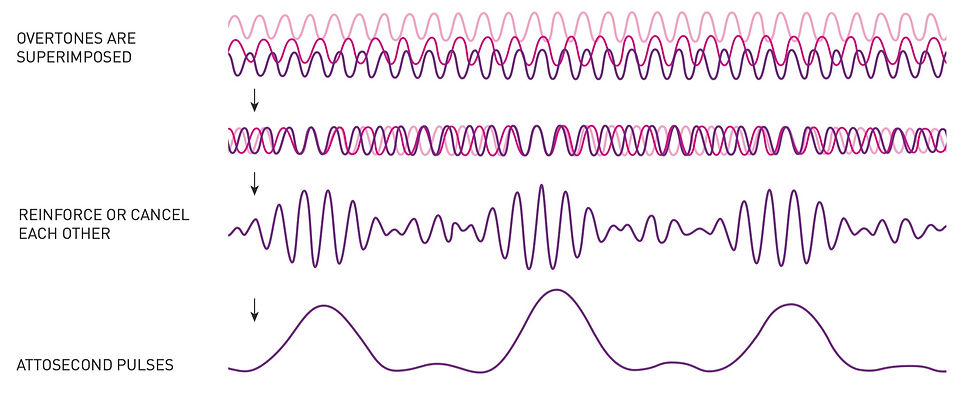
(Jarnestad & The Royal Swedish Academy of Sciences, 2023)
However, for some experiments, the constant stream of attosecond pulses might not be the most practical application, but rather it’d be better to have a single isolated attosecond pulse. In 2001, while Agostini made advancements on the former, so did Ferenc Krausz with the latter. To single out a lone attosecond pulse, Krausz’s group developed a similar method to Agostini’s work (also using constructive and destructive interference), in which the energy of the attosecond pulses are modified by another beam. This way Krausz could effectively produce a singular pulse lasting just 650 attoseconds (Hentschel et al., 2001).
The Future of Attosecond Physics
The creation of attosecond pulses allows physicists to study the interactions of electrons at an unprecedented level. Because the attosecond spectroscopy is so precise, “[it] can be used to nudge the electrons themselves, potentially eliciting a shift from passive observation to active control of chemistry on unprecedented scales” (Garisto, 2023). This is a monumental achievement. With a greater understanding of how electrons work, attosecond spectroscopy has a plethora of applications across all fields of science, specifically in electronics and medicine, which scientists are already looking into. It has subsequently started the field of attochemistry, where attosecond pulses are being used to control chemical reactions and explore the formation and breaking of chemical bonds (Castelvecchi & Sanderson, 2023). In 2010, Krausz used attosecond pulses to measure the speed of Einstein’s photoelectric, a process which, until then, scientists had assumed to be instantaneous (Schultze et al., 2010), and last year he used them to help identify subtle changes in blood as a possible precursor for early stages of cancer (Zigman et al., 2022). Another group used attosecond spectroscopy to find that the photoemissions of electrons in liquid water are 50 to 70 attoseconds faster than in its gas state (Jordan et al., 2020). All these new insights have revealed the tremendous potential in the technology. In an interview by Nature Photonics in 2022, L’Huillier says attosecond spectroscopy “[has] only scratched the surface”... “this field of research is evolving in a very promising way” (Pitruzzello, 2022).
References
cover image...
(Elmehed & Nobel Prize Outreach, 2023)

Comments